

Among Western musicians, it almost universally agreed upon that there is some harmonic quality called Consonance, and a negative to that called Dissonance; and yet there is almost no useful discussion regarding the nature of these two qualities. The various systems of Harmony which music theorists have constructed, from the Ars Noveau of the Middle ages to the modern Neo-Reimannian theories, have proven to be nothing more than classification systems. Harmony categorized by modes, Harmony categorized by function, Harmony categorized into Lewinian transformation - these are just systems which help us to recognize, imitate, and communicate harmonic patterns that we have already seen. They are not so much meant to be truthful as they are to be practical.
In the past couple of centuries, great advancements have been made in our scientific understanding of sound – that is, in the field of acoustics - but we have not seen any parallel advancements in our understanding of Consonance. This should tell us that the problems in our understanding are not due to a lack of facts, but due to the fundamentally flawed way in which we view them.
All music theorists have, without knowing it, subscribed to a very narrow conception of Harmony, a conception which was first formulated by Pythagoras and which, therefore, may be rightly called the "Pythagorean" view of Harmony. This conception can be summarized as follows:
1)All Harmony is composed of intervals.
2)The more numerically simple an interval is, the more Consonant it is.
In music, an "interval" describes the relationship between two pitches, usually in the form of a ratio. A pitch at 400 Hz and a pitch at 300 Hz, for instance, create the interval of 4/3. A pitch at 900 Hz and a pitch at 800 Hz create the interval of 9/8. According to the Pythagorean view of Harmony, the former is more consonant than the latter because the ratio between its two pitches is simpler.
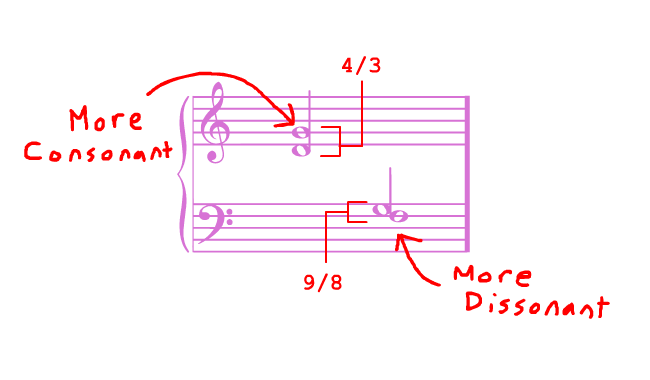
This way of thinking about Harmony has dominated Western music theory since its formulation 2500 years ago. Every one of the widely reknowned music theorists - Zarlino, Rameau, Reimann, Schenker, Partch, etc. - begins his Treatise of Harmony by listing off a set of intervals. Only once he has finished appraising each of these intervals as either consonant or dissonant does he then feel comfortable using them to reconstruct the classical scales and chords which he is used to.

The modern psychoacoustical approaches to music theory are also corrupted by this intervallic way of thinking. This much is clear from their Consonance-Dissonance maps, which all operate by mapping Consonance onto a domain of intervals:
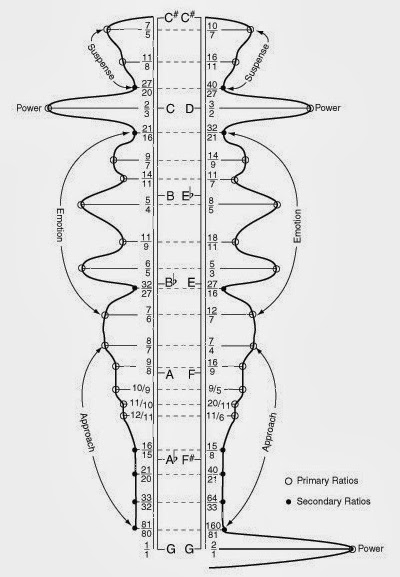

In both traditional and modern theories, then, the interval is always assumed to be the essence of Harmony. To music theorists, it is never the harmonic-structure ITSELF which is viewed as Consonant, but rather the intervals which it consists of. A major triad is not consonant; the 5/4 and the 6/5 within it are. The harmonic series is not consonant; just the intervals within it. Here is the core assumption which Pythagorean Harmony has implanted into the minds of theorists: that Harmony essentially only takes place at the level of the interval, and that the broader harmonic structures we have built - the chords, the scales, the songs - are merely sets of intervals shoved together. No matter how widely our conclusions about Consonance vary, they are always restricted from the very outset by this idea.
It doesn't seem to occur to anyone how narrow this conception of Harmony is. Has anyone, for instance, considered that there is no such thing as a "consonant interval?" Has anyone postulated the reverse of Pythagorean Harmony: that it might be the broader harmonic structure which is the true source of consonance, and that the intervals within this structure are not consonant, in themselves, but are just small fragments of a consonant whole?
The problems which confront music theory demand that we seriously consider such alternatives to Pythagorean Harmony and, moreover, that we broaden our vision of Harmony as a whole. We will never come to any new conclusions about the nature of Harmony if we continue to think of it in the same interval-centric way we have for the past 2500 years.
Before breaking away from this conception of Harmony, it would be worth tring to understand the areas in which it is lacking, so that we can fully understand which deficiencies have most plagued the field of music theory.
Underlying the Pythagorean view of Harmony is his philosophy: "the essence of all things is number." Like every truly great philosopher, Pythagoras' unconscious goal was not to understand the world, but to absorb it wholly into his own philosophy. In this case, the total absorption of the musical arts into his number-centric philosophy is probably the greatest victory of any philosopher in history.
Intervals are ratios, and ratios are numbers. In Pythagoras' purely intervallic music theory, number becomes the sole basis of Harmony. Even now, Harmony is something conceived of as purely numerical. So often, you hear people repeat that shallow aphorism, "music is math."
Of all the arts, only music has been subject to such a hyper-numerical treatment. When we look at a beautiful painting, like Rembrandt's "Night Watch," are we, at the bottom of it, just perceiving some mathematically perfect object - some formula or function which rendered into visual image? Absolutely not! The qualities which define the work - its colors, shapes, light, dark, shading, depth, framing, etc. - are all qualities particular to our sense of sight, qualities which cannot be reduced to mere number. Certainly, one could measure out some set of lengths in this painting, and one could argue that their "simple proportion" contributes, in some subtle way, to the piece's beauty, but only an absolute fool would claim that the entirety of the piece's aesthetic value rests on these proportions. But this is the exact treatment we have given music!
Compare, for a second, our descriptions of Harmony with our descriptions of other sensory phenomena. Our language for describing smells and tastes is almost entirely based on analogy: "fruitty," "nutty," "earthy," "salty," "spicy," - every one of these descriptors invokes an association with some actual sensual experience. Sight is described almost entirely in terms of colors, shades, and light - qualities which are specific only to that sense-world and which cannot be constrained by numbers. But even our words for colors were originally inseparable from the real-world objects which they were associated, as their etymological roots suggest: green with "grass" and "grow", yellow with "glow" and "gold," red with "reed" and "ruby" and "rhubarb." Basically, it is not far-fetched to assume that our words for colors originated in the same way as our words to describe tastes and smells, as references to actual visual objects, before gradually breaking away. Our language for describing Harmony lacks this kind of straightforward connection to the senses. Words such as "voice-like," "buzz-like," "howl-like," "chirp-like," "scream-like," "whisper-like," and "hum-like" are entirely absent from music theory, even though they would probably reveal far more about the meaning of a given harmony than the conventional "this chord consists of a 5/4, 5/3, 4/3, and 2/1."
Music, conceived of in this way, takes on an infinitely abstract character. Where other art forms like theatre, dance, and painting are viewed as sensuous, orgiastic, and intertwined with life itself, music is thought to be as abstract, cold, and logical as the numbers which supposedly make it up. People would like to believe that music is this intangible, up-in-sky thing And this is part of the reason the Pythagorean conception persists: it provides a simple but highly conceptual answer to the questions about Harmony, so that we don't really have to ask any more questions about it. All of the questions about music can be covered when one casts the broad conceptual net, "music is math." One hears that shallow aphorism everywhere, and people just seem to completely satisfied with it. Why is Harmony beautiful? "Because we're like... hearing numbers." What is so beautiful about the numbers? "Ummm, they just ARE, okay?!!"
So, due to the unchecked influence of Pythagorean Harmony, a great divide has been placed between our understanding of Harmony and our basic sensuous impressions. It seems to me that this is precisely what has atrophied our music theory: this uprooting of our theories from the soil of the sensory, the real, and the actual.
In this blog, I would like to propose a theory of Consonance which has its basis, not in numbers, but in actual sounds. We reject the Pythagorean view of Harmony which, by its callous reduction of Harmony to a purely numerical form, has distorted our impressions of music so that the average person ocan only think of it as an artform dominated by logic, patterns, and measurements. In contrast to this Pythagorean conception, we view music to be illogical, strange, and amorphous. We take music for what it is. Music is sound, and the former will never be understood if we do not thoroughly understand of the latter. Every numerical representation of Harmony, therefore, will be treated with the utmost suspicion, and every attempt will be made to understanding harmonies by drawing analogies to the basic sounds which we are most accustomed to. In this way, we hope to re-establish the connection between our music theory and our basic sense-perceptions which has long been severed.
All sound is made up of frequencies, oscillations of air pressure over time. Frequencies should be regarded as atomistic to the world of sound, in that they are the indivisible sound particles which by themselves mean little but out of which every sound can be produced. On the other hand, to say that the sound-world is “just a bunch of frequencies” would be a reduction as shallow and useless as saying that the physical world is “just a bunch of atoms.”
What matters is clearly not the frequency-atoms themselves, but the shapes which are made when they combine together. Out of the infinite variety of these shapes, I distinguish between two fundamental types: Tone and Noise.
A tone is a sound which is made of many frequencies but is heard as one pitch. Noise is a sound which is composed of many frequencies but is heard as having no pitch.
The distinction I make between frequency and pitch, here, may confuse some readers, as the two terms are almost always used interchangeably. Conventionally, it is understood that a physical frequency at 400 Hz will be perceived by the listener to be at the pitch of 400 Hz, end of story. But this fact only applies to frequencies in isolation. When we hear multiple frequencies at once, there is almost always a disparity between the actual number of frequencies, and the number of pitches we perceive in them. This disparity is most clearly exemplified in Tone and Noise.
This is the frequency spectrum of the sound produced when a C3-note on a piano is played:
This is a tone. We have multiple frequencies playing simultaneously, but rather than hearing many pitches, as one might expect, we only hear one. Every tone that we hear, whether its source is a piano, violin, human-voice, didgeridoo, bird-call, or trumpet, is, like the tone above, a collection of frequencies which combines together to create a single pitch. This is a fact which often suprises the uninitiated into acoustics, because the pitch created by the set of frequencies is so absolute and so pure.
On the other hand, here is the frequency spectrum of the sound produced by a cymbal crash:
This is a noise. Once again, we have multiple frequencies playing simultaneously, but this time we do not perceive any single pitch; rather, we perceive a general range of pitchless sound.
So, in these two sound-types, the separateness of "frequency" and "pitch" is made clear: frequencies are the physical components which make up every sound, but pitch is a feeling resulting from the arrangement of these frequencies. Going forward, the reader will find that much of Harmony depends on this unequal relationship between pitch and frequency.
And so, keeping in mind the distinction between frequency and pitch, we can begin to understand the first of our two types of sound: Noise.
Noise is sound-without-pitch. If we hear a frequency in isolation, we register it as a pitch, as a single "point" in acoustical space. But the more frequencies we hear simultaneously, the more difficult it becomes to identify them as individual pitch-points. Put simply: the more frequencies we hear, the more they "clutter" our range of hearing.
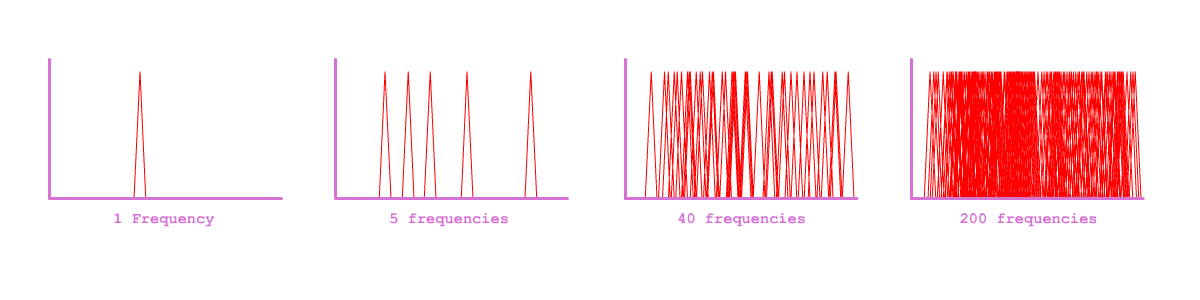
At some point, we cease to register individual pitches at all, instead perceiving a range of pitches. This is roughly where a sound becomes a NOISE. Just as we would perceive thousands of grains of sand not individually but as a single homogenous substance, so too do we experience thousands of frequencies not individually but as a single, homogeneous noise. Rather than appearing as distinct harmonic identities, each frequency fades into the next, forming a continuum of sound. And so, noises are formless, indefinite blurs of sound.
Whereas a tone is defined by the particular placement of its frequencies, a noise is defined only by the general range that they occupy. The former is sound-as-structure, whereas the latter is sound-as-mass.
"Brown" noise (frequencies occupying the lower range of human hearing):
"White" noise (frequencies occupying the full range of human hearing):
"Purple" noise (frequencies occupying the higher range of human hearing):
Noise is the lowest, most fundamental state of sound. Much like a cloud is just a temporary almagam of various water-particles, a noise is really just cluster of disjointed frequency-particles which, merely by their proximity to each other, appear as a single entity. Wherever we delineate the beginnings and ends of this cluster will be totally arbitrary. Two noise-clouds will not be heard as separate; they will bleed into each other, combining into a larger frequency-mass. Noise, then, is sound-without-form.
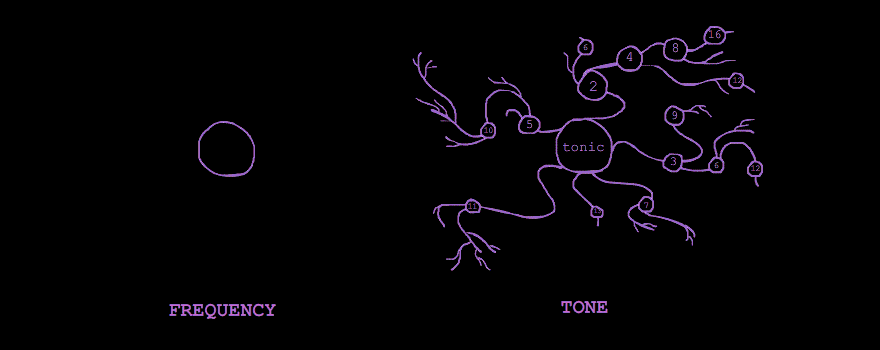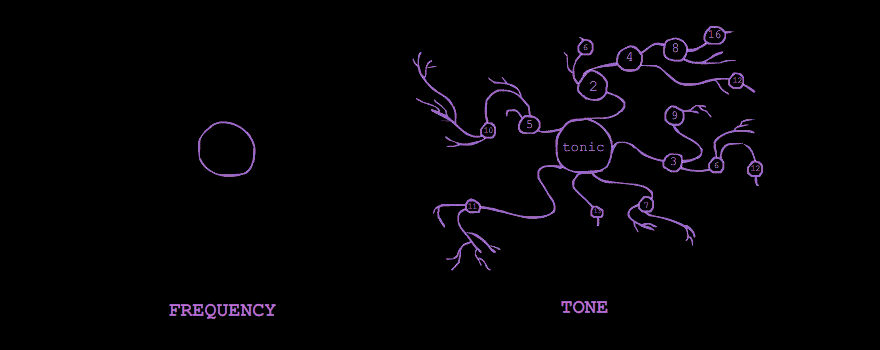
Whereas Noise is sound-as-mass, the Tone is sound-as-structure. A tone's frequencies fit a very specific arrangement which allows them all to combine into a singular pitch-feeling. This structure, the anatomy underlying every tonal body, is the Harmonic Series.
The Harmonic Series - sometimes called the "overtone series" - is a series of frequencies naturally created by the resonations of a physical body. If a piano-string, for instance, were to vibrate at a certain frequency, this frequency would resonate with the rest of the piano's body, and these subsequent resonations would produce a series of higher frequencies which would all be multiples of the initial frequency. If a string vibrates at 100 Hz, for instance, it will create a series of frequencies at 200 Hz, 300 Hz, 400 Hz, 500 Hz, etc.
This naturally occuring frequency spectrum is the Harmonic Series. Each frequency in this Series is called a "harmonic," and the lowest of these harmonics, the initial frequency, is called the "tonic." The tonic determines the pitch that we hear, and every other harmonic assimilates into this pitch. Heard as a whole, this collection of frequencies makes up the sound I call "the Tone."
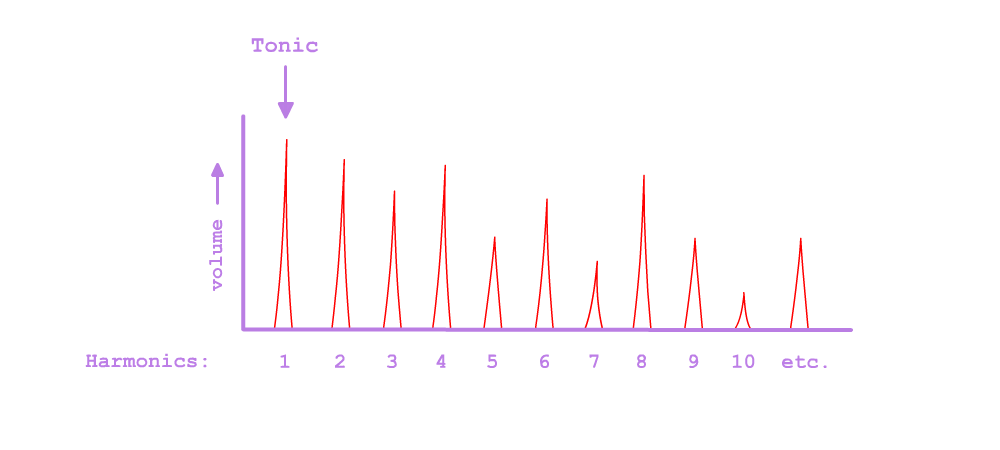
What makes the Tone altogether different from Noise is that it is a structure of frequencies rather than just a haphazard cluster of them. Within a single tone, every frequency bears a unique relation to a common pitch. The 2nd harmonic, the 3rd, the 5th, the 7th, etc. - each one assimilates uniquely into the pitch-feeling decided by the tonic; each forms a unique "limb" in an over-arching acoustical body. The 3rd and the 5th harmonic might both assimilate into the same pitch, but each adds its own particular "flavor" to the mix. It is by virtue of their positions in an over-arching structure, therefore, that each harmonic gains a unique identity. So, each tone conceals great inner-complexity. What we hear to be a single pitch is, in actuality, a sprawling network of interrelated harmonic identities.
This network, if the reader will allow me to draw a blatantly visual metaphor, can be described as such: the pitch is something akin to a nucleus, a central point which defines the position of the body. Every other harmonic, from this central point, extends outwards into something akin to a "shape."
This outward "shape" which is formed by the harmonics is called Timbre. Timbre is basically the whole of harmonic expression. A pitch is just a point in harmonic space, but Timbre is the shape which forms around this point - the tone's breadth and length; its faces and sharp edges. We can hear two tones which are at the same pitch, but still experience two entirely different harmonic feelings by virtue of their different timbres.
Though the existence of the Harmonic Series is tacitly accepted by theorists, the deeper implications of this natural sound-structure and the way we hear it remain largely ignored. Every tone that we hear, whether it comes from a piano, trumpet, didgeridoo, bird, or human voice, has a harmonic series. Music theorists generally still treat every tone as a single "pitch-class," but the existence of the Harmonic Series should dissuade us from reducing the Tone to just a pitch. Every tone is composed of many frequencies. The Tone is, itself, a harmonic structure!
This is a single frequency in isolation:
But THIS is a tone:
There is a world of difference between the two! Both are heard as a single pitch, but, while the frequency is just an acoustical "atom," the Tone is an elaborate acoustical molecule - one in which many interrelated frequencies contribute to a single over-arching feeling of sound.
Although the Tone is a structure made up of several frequencies, we only hear this collection of frequencies as a single pitch. No other harmony can boast this level of unity. Every sound is really just a collection of frequencies, but only in the Tone do these frequencies interlock together so seamlessly that we fail to distinguish between them entirely.
These facts lead us to a conclusion which should have been obvious from the beginning: The Tone is the only pure consonance.
This is an idea which goes overlooked only because of the persistence of Pythagorean theory, which has led music theorists to only look at the intervals between tones, rather than the tones themselves. But, once we ditch the narrowness of the Pythagorean theory, it becomes obvious that the Tone epitomizes every quality which belongs with Consonance. In the Tone acoustical unity is absolute. Every harmonic is perfectly assimilated into a single feeling of pitch. The Tone lacks any of that desire to "resolve" which is so indicative of dissonances. The Tone is a perfect harmony.
These two fundamental sounds, Tone and Noise, represent the furthest extremes of Harmony. The Tone is one-pitched sound; Noise is sound-without-pitch. 1 and 0. The form and un-form of our sound-world. The whole of our acoustical experience rests upon the distinction between these two extremes.
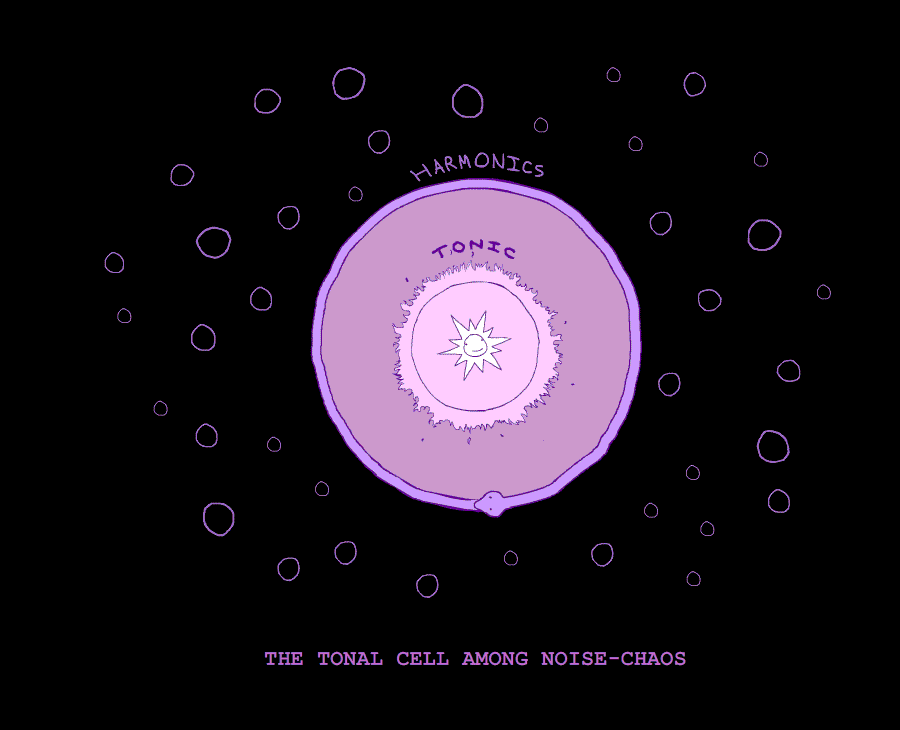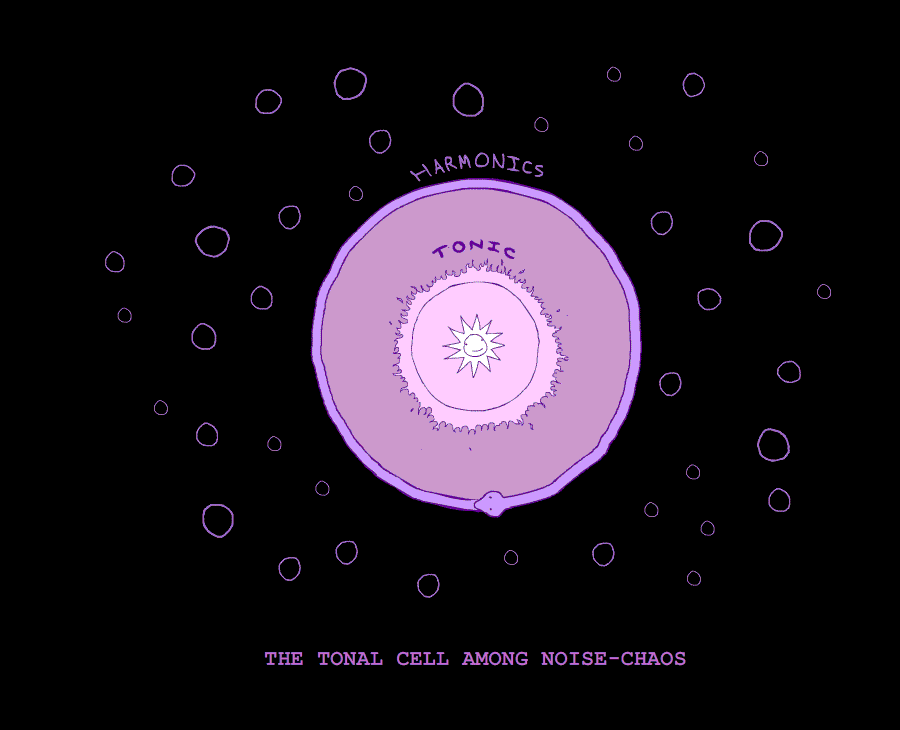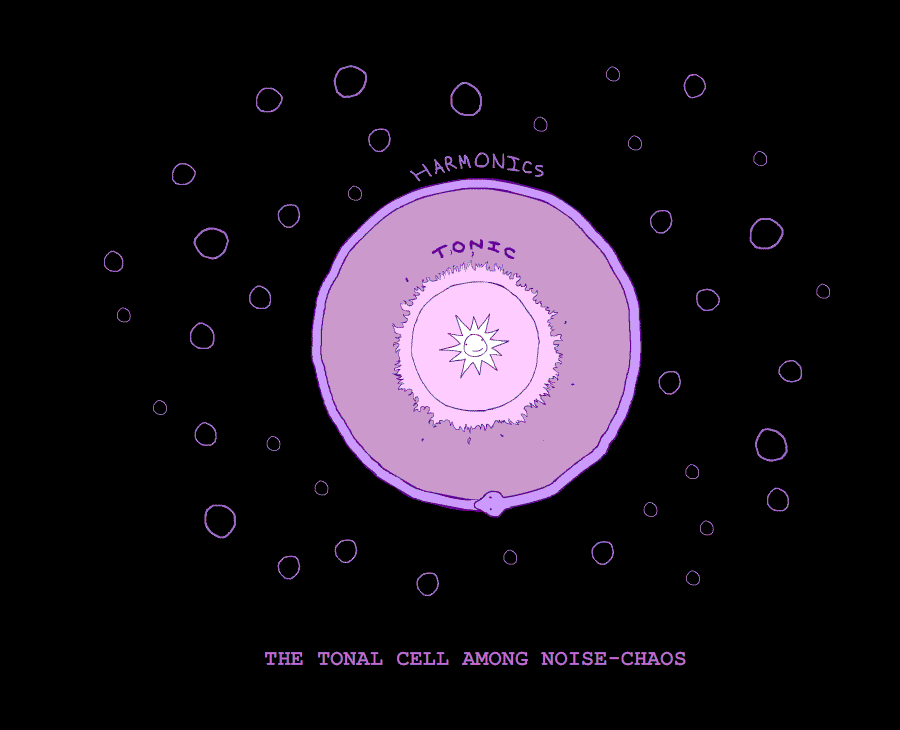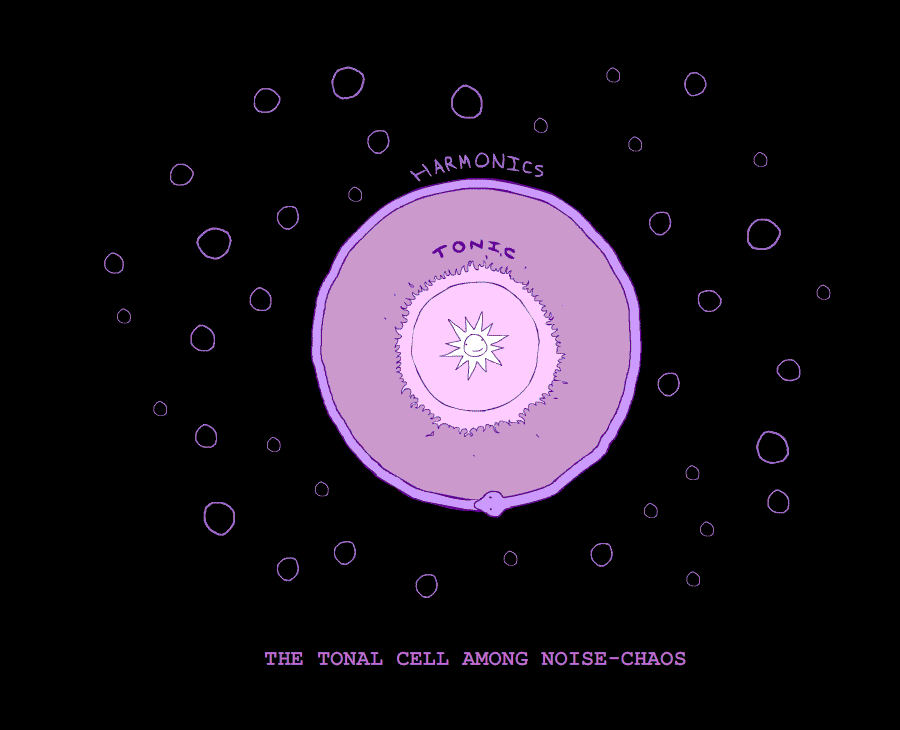
To truly appreciate the enormity of Tone and Noise within our acoustical experience, one has to actually be outside, away from the intrusion of public speakers (always growing more and more inescapable as time goes on), and take note of the sounds which reach the ear. One will soon find that all of these sounds can be neatly categorized into either a tone or a noise. The buzzing of cicadas, the blowing of wind, the rustling of leaves, the rolling of tires along pavement - these are all noises, blurs of pitchless sound. The singing bird, the chattering person, the car horn - each of these is a tone, a single fluctuating pitch buffered by a harmonic series. THIS is the world of sound in its purest, most untouched state, before the majority of people felt it necessary to fill every public space with incessant background music (totally against the will of those who enjoy their internal monologue). This is the acoustical environment within which our ear developed and according to which it transformed itself. There were no intervals, no perfect-fourths, no perfect-fifths, no major triads; only Tone and Noise poised in violent contrast to one another.
In the sound-world, Noise is almost omnipresent. There is no silence in our world; only a vague undercurrent of pitchless sounds. The buzzing of cicadas, the blowing of wind, the rustling of leaves, the rolling of tires along pavement - these are all noises, pitchless waves of sound bleeding into each other, becoming. The world of sound, by default, is a boundless ocean of noises. Tones, by comparison, are fleeting and rare. They appear briefly amidst Noise, like waves upon the surface of a vast ocean, rising up and sinking back into its overwhelming mass. So, every tone juxtaposes itself to the ceaseless stream of noises which flow underneath, behind, around it. The world of sound, at its core, is a timeless landscape of noises upon which many tonal-voices blossom outwards, always appearing briefly and then decaying back into Noise's ever-shifting mass.
Nowhere else is the duality between Tone and Noise more clearly expressed than in our speech. Human speech always consists of a quick fluctuation between various Tone-timbres and Noise-ranges. "Ah," "Eh," "Ee," "Oo" and "Oh" are all tonal sounds which we distinguish according to their different timbres. "Ch" "Ss" "Tuh" and "Puh" are all noises which we distinguish according to their different ranges. In speech, therefore, we witness a prototype of Harmonic progression, a sequential ordering of the two basic sound-types, and a pushing of each one to the expressive limits allowed by the human voice.
The dichotomy between Tone and Noise, then, is as fundamental to the ear as the dichotomy between Light and Dark is to the eye, and equally rife with spiritual meaning. Our ear is not, as theorists are so eager to believe, a cold and mechanical interval-calculator; our ear simply recognizes the basic sound-forms that it evolved alongside. It does not measure out;it recollects.
My answer to the Consonance problem is simple: Consonances are Tone-like sounds; dissonances are Noise-like sounds.
The sensory phenomenon of "Consonance" arises from our necessity to detect tonal voices out in nature and to distinguish them from a constant stream of background noises. This capacity for Tonal detection grows to be particularly sophisticated in humans in response to the complexity of our speech, which requires us to quickly and accurately discern between a wide range of timbres (tonal expressions). The difference in timbre between "Ah" and "eh" is minute, but this
Consonant "chords" are possible because many tones can fit together in such a way that they create the impression of a singular tone. This is, essentially, the
Say that a church-organist is having an off-day, and he accidentally starts the hymn by playing this chord:
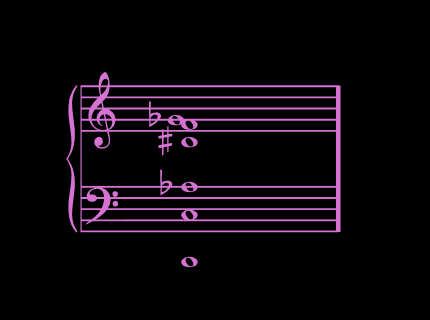
This sound is a clear dissonance. It consists of 6 separate tones - 6 ENTIRELY DIFFERENT PITCHES which fighting for the ear's attention. We have, then, a cluster of pitches which, heard together, are Noisy.
So, quickly fixing his mistake, the organist voice-leads to a strong consonance, a towering C-major chord:
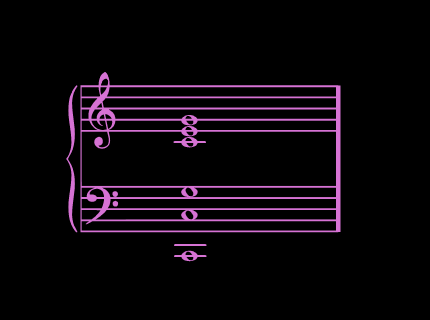
Just by slightly shifting each of these pitches, the organist has eliminated that unsettling feeling of 6 pitches fighting against each other. But why? Why do these 6 tones feel unified whereas the previous 6 tones do not? It is because, although the organist is technically still playing 6 tones, all of these tones conform to a single Harmonic Series!
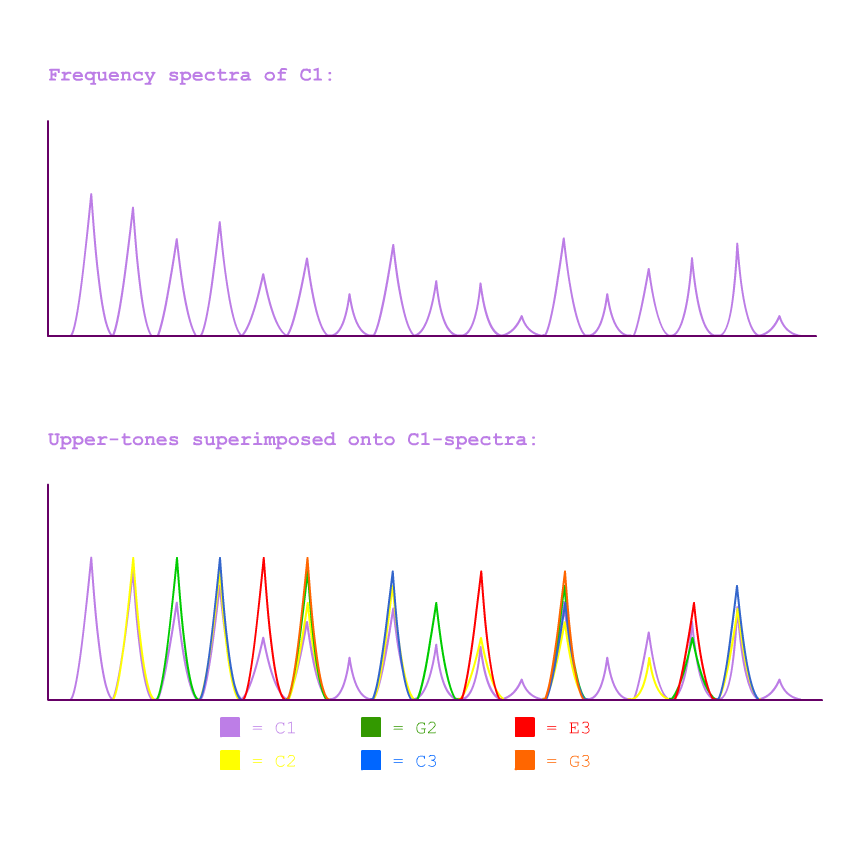
When multiple tones fall along a single Harmonic Series the resulting sound tricks the ear. Our ear recognizes not the individual tones but the over-arching Series which they fall into. As a consequence, rather than hearing 6 separate tones, we perceive a single Tone-like sound! The 6 pitches no longer conflict with each other, because each one is incorporated into the harmonic series of C1. C2, for instance, becomes the 2nd harmonic of C1's series. C5, likewise, becomes C1's 5th harmonic. Each of the 5 upper-tones is assimilated into the C1-tone. What we get, as a result, is a single network of frequencies stemming from the pitch of C1.
The most widely accepted psychoacoustical explanation for Consonance is Helmholtz's theory that Consonance is the direct result of overlapping harmonics. But this is only a half-explanation. It doesn't get to the core of what's really happening. What Helmholtz misses is the fact that whenever 2 separate harmonic series overlap with each other, they necessarily create a larger kind of meta series through their union:
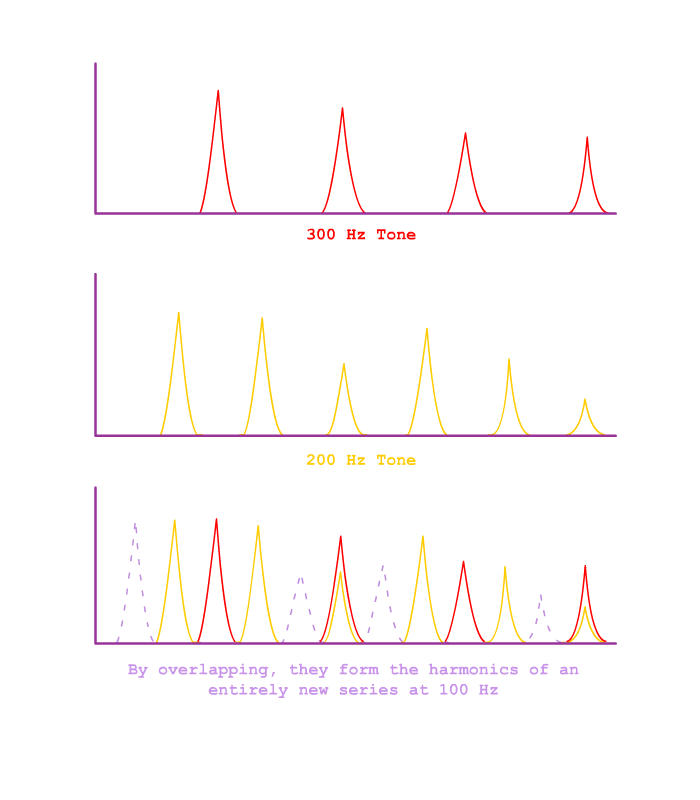
It is by virtue of this "meta" series that the tones unify. The source of consonance is not in the two actual tones, but in the Tone-like sound which is created by their union. So, it is not that the tones are "consonant with each other," as such; rather, by their union, they become ONE TONE!
So, Consonance is not a matter of number, but of semblance. A sound may not be a pure tone, but it might be similar enough to a tone to at least rouse our Tone-seeking ear - to make it turn its head and go "huh? wuh?" Just as when a cloud resembles a human face, even in the slightest way, it naturally draws the focus of the eye, achieving distinction against the formless sea of clouds surrounging it, in a similiar way does a sound which even slightly resembles a tone draw the focus of the ear, achieving distinction, in this case, against a formless sea of noises. Chords and intervals can be Consonant on this very basis. The extent of any sound's consonance can be determined simply by comparing it to a pure tone. The more closely a sound resembles the Tone, the more Consonant it is; the more a sound deviates from the sound of the Tone, the more dissonant it is.
Tonality, then, is not something peculiar to Western Harmony, but a principle which is common to all styles of Harmony. Wherever one finds a Tradition of Harmony, whether it is Islamo-Indian Raga, American Jazz, or any of the thousands of locally-bound folk musics lying scattered across the globe, one will always find some elaboration upon the Tone. All sound-structure is Tonal, and the properties inherent to this structure are something which every composer must deal with, just as every architect must deal with the laws of gravity. This is a fact not consciously realized but instinctually felt by every composer, every tuner, and every performing musician. Everyone who has ever contributed to the progression of their own musical culture has, without realizing it, felt the pull of the Tone upon his ear. The sense for Tone-like sound, hammered into his psyche by millions of years of evolution, unconsiously influences every musical decision he makes, driving him to create scales, chords, progression, melodies, and songs which ground themselves in the firm structure of the Tone. For the moment he strays too greatly from this structure, he invokes Noise, and, with it, the grey and banal feeling of acoustical homogeneity.